The Zone of Proximal Development in the classroom and on HappyNumbers
In this article we’ll discuss the Zone of Proximal Development, its importance for classroom instruction, and how we apply this concept within Happy Numbers.
Try Happy Numbers (it’s free to try!) and watch your students grow 1.5 grade levels in just one school year!
What is ZPD?

Let’s first imagine a typical classroom situation: Mary is a 1st grade student. She is good at mental addition and subtraction of numbers to 20 without crossing a ten (12 + 5 = ?), but struggles every time she needs to add across ten (8 + 7 = ?). She could have solved this problem using counting on, but this method takes too much time and often leads to mistakes. The “making a ten” method definitely seems to be a gap for her.
In today's lesson, Mary’s teacher introduced the addition of numbers to 40 with and without crossing a ten. Attention was focused on operating with greater tens, and the “making a ten” method was considered as already mastered. Mary realized that this was too hard for her and felt frustrated.
What happened here can be explained in terms of the ZPD concept. The new topic (addition to 40 with and without exchange) was not proximal to Mary, or in other words, it was something she couldn’t do without assistance provided.
In general, for a student to learn in the ZPD, we should offer a problem that is:
- not too hard, so that he or she could do it with a moderate amount of assistance from the teacher or other resources;
- not too easy, so the problem brings out some new concepts for the student.
- not too hard, so that he or she could do it with a moderate amount of assistance from the teacher or other resources;
- not too easy, so the problem brings out some new concepts for the student.
This will let the student work at his or her comfort level with maximum motivation.
Empirical observation of ZPD
The ZPD concept is a powerful and thoughtful theory, developed by Lev Vygotsky at the beginning of the 20th century. Unfortunately, Lev Vygotsky lived a short life (1896 - 1934) and left many questions unanswered. One of them is, How can we empirically observe ZPD? Since then, numerous experiments have been conducted to answer this question.
One of them (C. Ruiz-Hernández, 2017) [1] was designed as an arithmetic worksheet offered to a group of as many as 80 college students. The questionnaire contained a pair of problems, which authors labeled as P and P’, where P’ was relatively more difficult than P. Half of the students (the control group) got the test without any clues, while the other had a clue in the heading to problem P’ (experimental group). All students first attempted problem P and then proceeded to P’.
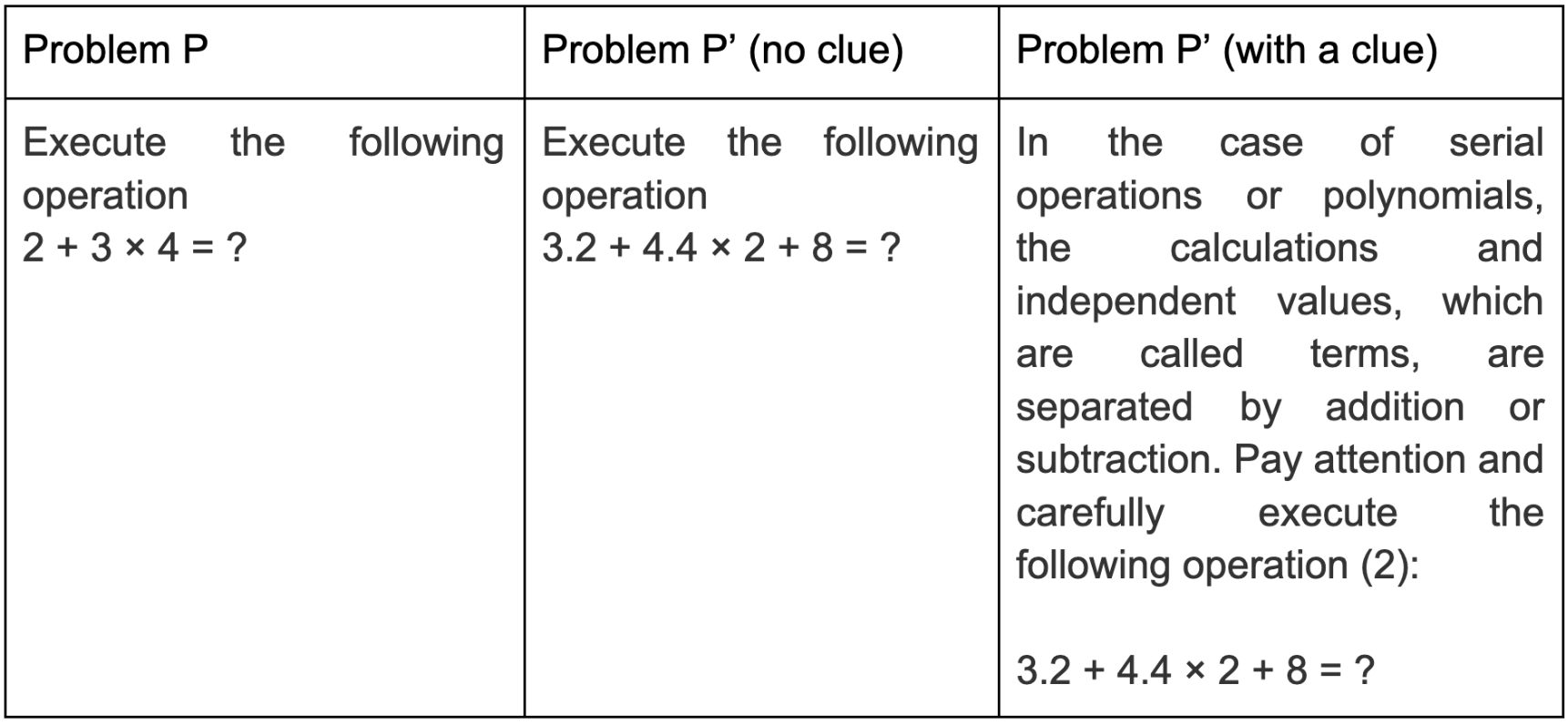
In the control group (with no hints provided), there were 10 students who solved the harder problem (P’), and all of them got the easier task (P) correct as well. There were no students in this group who struggled with the easier problem (P) but solved the harder one.
In the experimental group (which had a hint to the more difficult problem), 19 students solved the harder problem (P’), and only 9 of them got the easier one (P) correct. Thus, the authors conclude that 19 - 9 = 10 students took the advantage of the help provided. This result enables us to empirically observe the phenomenon similar to the ZPD in the context of a test. Namely, we observe that for these 10 students the harder problem (P’) was in their ZPD: they could not solve it independently, but managed to do it with a hint.
Application of ZPD in the classroom
Another question that bothers the researchers is how the concept of ZPD may be applied to classroom instruction.
There are two key points here:
1. Providing assistance for students when learning a new concept. It can be done in several ways, such as:
1. Providing assistance for students when learning a new concept. It can be done in several ways, such as:
a. Formulas or key words written on the board while discussing a challenging problem;
b. Posters or illustrations placed around the classroom;
c. Printables with a plan of action, models, or answer structure;
d. Direct teacher guidance;
e. Peer-to-peer interaction.
2. Constantly monitoring each student’s level followed by further material adaptation and applying different teaching techniques, so that each student stays within his or her ZPD. This can be done with the help of:
b. Posters or illustrations placed around the classroom;
c. Printables with a plan of action, models, or answer structure;
d. Direct teacher guidance;
e. Peer-to-peer interaction.
2. Constantly monitoring each student’s level followed by further material adaptation and applying different teaching techniques, so that each student stays within his or her ZPD. This can be done with the help of:
a. Assessments;
b. Analysis of practice work;
c. Open discussions in the classroom.
b. Analysis of practice work;
c. Open discussions in the classroom.
As a core of the ZPD, the idea of a teacher's constant assistance has also been a common topic for discussion among scientists. A number of experiments have considered the effect of different ways assistance can be provided in the classroom. Here are some important points that a teacher may find useful for his or her practice:
- If no kind of feedback is provided, then working in pairs is more beneficial than working alone, particularly for those students who are paired with a more competent partner.
- However, more advanced students might improve while sharing understanding with lower-level partners, but in some cases these “expert” students may regress, particularly if a lower-level partner is confident in her/his error solution. Feedback from the teacher or other materials (clues or models) are significant in preventing the higher-level student from regression.
- All in all, most researchers agree that student collaboration may be beneficial under certain conditions, but some of them insist that appropriate feedback from a teacher or the materials (if available) have a greater influence on the effect of the learning process in the ZPD.
- However, more advanced students might improve while sharing understanding with lower-level partners, but in some cases these “expert” students may regress, particularly if a lower-level partner is confident in her/his error solution. Feedback from the teacher or other materials (clues or models) are significant in preventing the higher-level student from regression.
- All in all, most researchers agree that student collaboration may be beneficial under certain conditions, but some of them insist that appropriate feedback from a teacher or the materials (if available) have a greater influence on the effect of the learning process in the ZPD.
The role of mistakes
So, feedback is a great instrument for effective learning in the ZPD, and the way adults react to student mistakes is just as important.
Children who get negative feedback may start to avoid any situation in which they can make a mistake. Therefore, they won’t attempt a problem that is proximal to them until they get strong assistance from the teacher or other resources.
To support a struggling student, a teacher may:
- Admit that making a mistake indicates that the student is really learning, which is great. We can evaluate the level of brain activity judging by the mistakes we make. In fact, the brain really develops new connections mostly when we struggle with solving difficult tasks.
- Move the emphasis in the feedback from points lost to recommendations that should be followed to improve.
- Recognize each (even minor) improvement that has taken place.
- Admit that making a mistake indicates that the student is really learning, which is great. We can evaluate the level of brain activity judging by the mistakes we make. In fact, the brain really develops new connections mostly when we struggle with solving difficult tasks.
- Move the emphasis in the feedback from points lost to recommendations that should be followed to improve.
- Recognize each (even minor) improvement that has taken place.
ZPD in HappyNumbers
Happy Numbers is designed to provide each student with a learning experience that lies within his or her ZPD. There are several ways in which we determine it and incorporate it into our lessons.
Placement Test
Upon entering Happy Numbers, all K-5 students automatically take the Happy Numbers Placement Test. There is no placement test in PreK at the beginning of the school year, since these students will all start their learning pathway from the beginning of the curriculum.
The test consists of a wide range of problems that adapt to the student’s level as he goes through it. The results of the test show a student's current readiness for mathematics instruction depending on grade-level skills and concepts. It also helps place the student at the appropriate starting point in the Happy Numbers curriculum and create an individual learning pathway for him or her.
In other words, we place the student in her/his ZPD or a little before that, and our responsive technology provides all the necessary assistance needed to improve his or her skills.
Click for additional information about the Placement Test on Happy Numbers.
Mid-Year Test
Each student progresses through the curriculum at an individual pace and has his or her own unique struggles while learning. The Mid-Year Test was designed to adjust the learning pathway by determining the topics the student has mastered more quickly than the curriculum suggests. These topics will be skipped to let the student focus on what is in her/his ZPD at the moment.
Feedback from interactive model
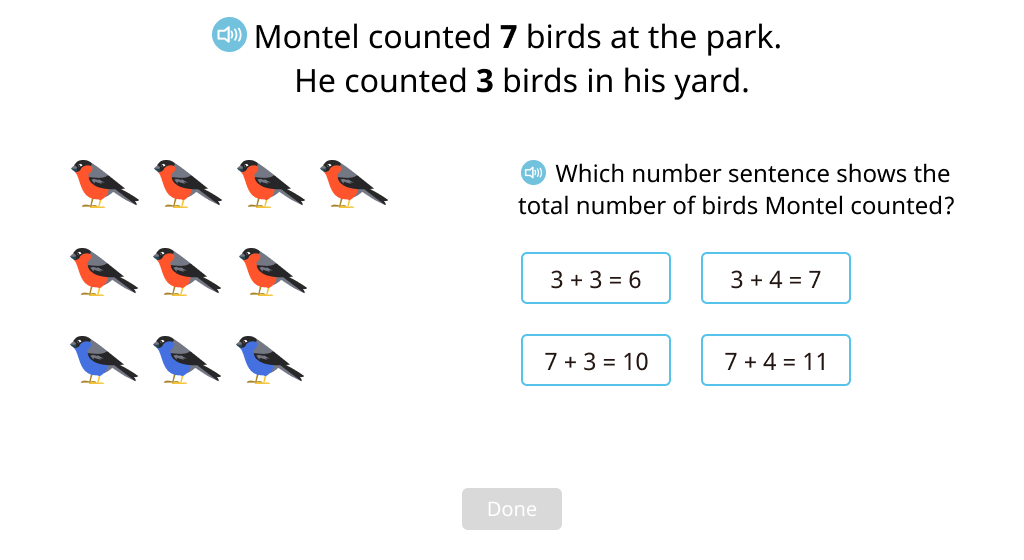
We’ve seen that appropriate feedback is extremely important while learning in the ZPD. Such feedback may be provided by the problem itself, if it contains a picture or a model students can interact with to check understanding. Like in this Happy Numbers’ exercise where students have to manipulate the shapes to represent a given portion:
Hints and Let’s do it together
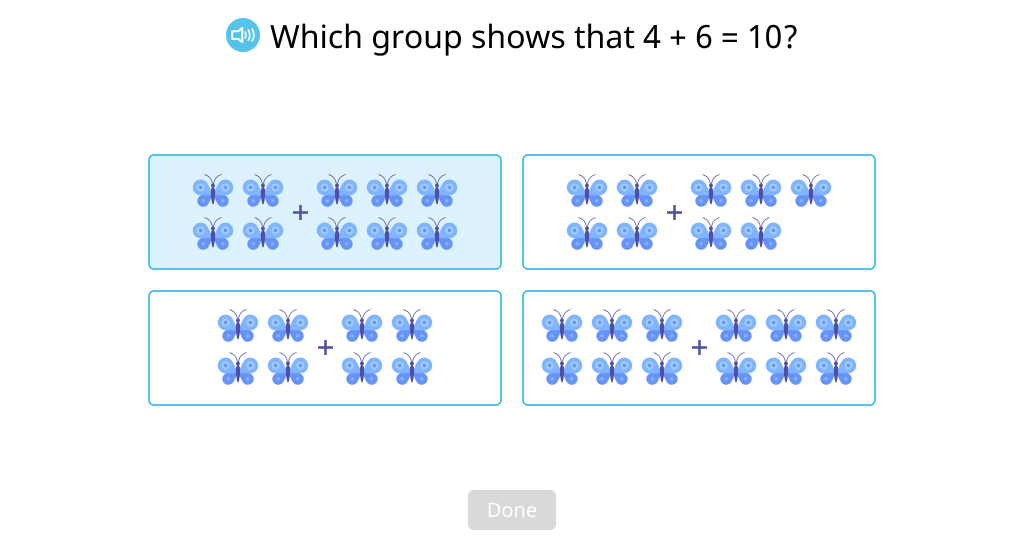
If students struggle with a problem on Happy Numbers, they receive different levels of assistance depending on their personal needs.
A student doesn’t stay one-on-one with his or her misunderstanding. Happy Numbers will provide a comment, a hint, or additional animation to support the student. If this is not enough, we will simplify the problem by dividing it into several steps and move through the solution together.
Below is the example of how Happy Numbers helps to find neighboring hundreds for a number, first, showing a number line, and then giving detailed instructions on how to work on it.
Below is the example of how Happy Numbers helps to find neighboring hundreds for a number, first, showing a number line, and then giving detailed instructions on how to work on it.
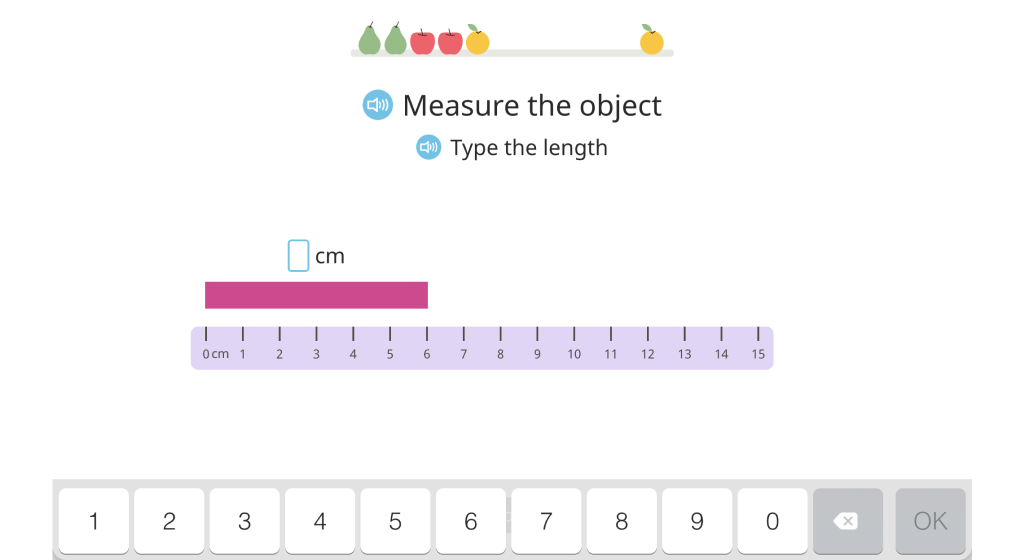
Beads system
To ensure that a student moves on to the next concept only after he or she has mastered the previous one, Happy Numbers uses a clear and visual scoring system. As shown below, there are green beads (or fruits) at the top of the screen for every task. When a student enters a correct response, one green bead moves from left to right on the counter. If a student enters an incorrect response, one bead moves from the right back to the left. The goal is to move all the beads from the left to the right to advance to the next task.
Therefore, a visual scoring system helps adapt the number of problems a student must solve correctly to show mastery.
Voiceover
For students who can’t read fluently yet, the text might prevent them from learning in their ZPD even if the math concept is reachable. For such students, a natural human voiceover is available on all PK-5 tasks in Happy Numbers.
Resources:
[1] Ruiz-Hernández, C.; Lupercio Lozano, A.; Bernal González A. (2017).
Towards an Experimental Verification of Vygotsky's Zone of Proximal Development: A Docimological Approach. Education Journal. 6(1): 47-50
[1] Ruiz-Hernández, C.; Lupercio Lozano, A.; Bernal González A. (2017).
Towards an Experimental Verification of Vygotsky's Zone of Proximal Development: A Docimological Approach. Education Journal. 6(1): 47-50